So, we have powers of two. If you take the number from the bottom line, you can easily find the power to which you will have to raise two to get this number. For example, to get 16, you need to raise two to the fourth power. And to get 64, you need to raise two to the sixth power. This can be seen from the table.
And now, actually, the definition of the logarithm:
The base a logarithm of x is the power to which a must be raised to get x.
Notation: log a x = b, where a is the base, x is the argument, b is what the logarithm is actually equal to.
For example, 2 3 = 8 ⇒ log 2 8 = 3 (the base 2 logarithm of 8 is three because 2 3 = 8). With the same success, log 2 64 = 6, since 2 6 = 64.
The operation of finding the logarithm of a number to a given base is called logarithmization. So, let's add a new line to our table:
| 2 1 | 2 2 | 2 3 | 2 4 | 2 5 | 2 6 |
| 2 | 4 | 8 | 16 | 32 | 64 |
| log 2 2 = 1 | log 2 4 = 2 | log 2 8 = 3 | log 2 16 = 4 | log 2 32 = 5 | log 2 64 = 6 |
Unfortunately, not all logarithms are calculated so easily. For example, try to find log 2 5. The number 5 is not in the table, but logic dictates that the logarithm will lie somewhere on the interval. Because 2 2< 5 < 2 3 , а чем больше степень двойки, тем больше получится число.
Such numbers are called irrational: the numbers after the decimal point can be written ad infinitum, and they are never repeated. If the logarithm turns out to be irrational, it is better to leave it that way: log 2 5, log 3 8, log 5 100.
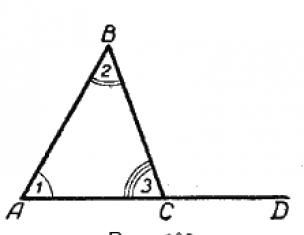
It is important to understand that a logarithm is an expression with two variables (the base and the argument). Many people at first confuse where the basis is and where the argument is. To avoid annoying misunderstandings, just look at the picture:
[Caption for the picture]
Before us is nothing more than the definition of a logarithm. Remember: logarithm is a power, into which the base must be built in order to obtain an argument. It is the base that is raised to a power - it is highlighted in red in the picture. It turns out that the base is always at the bottom! I tell my students this wonderful rule at the very first lesson - and no confusion arises.
We've figured out the definition - all that's left is to learn how to count logarithms, i.e. get rid of the "log" sign. To begin with, we note that two important facts follow from the definition:
- The argument and the base must always be greater than zero. This follows from the definition of the degree rational indicator, to which the definition of a logarithm comes down.
- The base must be different from one, since one to any degree still remains one. Because of this, the question “to what power must one be raised to get two” is meaningless. There is no such degree!
Such restrictions are called range of acceptable values(ODZ). It turns out that the ODZ of the logarithm looks like this: log a x = b ⇒ x > 0, a > 0, a ≠ 1.
Note that there are no restrictions on the number b (the value of the logarithm). For example, the logarithm may well be negative: log 2 0.5 = −1, because 0.5 = 2 −1.
However, now we are only considering numeric expressions, where it is not required to know the logarithm's CVD. All restrictions have already been taken into account by the authors of the problems. But when they go logarithmic equations and inequalities, DHS requirements will become mandatory. After all, the basis and argument may contain very strong constructions that do not necessarily correspond to the above restrictions.
Now let's consider general scheme calculating logarithms. It consists of three steps:
- Express the base a and the argument x as a power with the minimum possible base greater than one. Along the way, it’s better to get rid of decimals;
- Solve the equation for variable b: x = a b ;
- The resulting number b will be the answer.
That's all! If the logarithm turns out to be irrational, this will be visible already in the first step. The requirement that the base be greater than one is very important: this reduces the likelihood of error and greatly simplifies the calculations. It’s the same with decimal fractions: if you immediately convert them into ordinary ones, there will be many fewer errors.
Let's see how this scheme works using specific examples:
Task. Calculate the logarithm: log 5 25
- Let's imagine the base and argument as a power of five: 5 = 5 1 ; 25 = 5 2 ;
- Let's create and solve the equation:
log 5 25 = b ⇒ (5 1) b = 5 2 ⇒ 5 b = 5 2 ⇒ b = 2; - We received the answer: 2.
Task. Calculate the logarithm:
[Caption for the picture]
Task. Calculate the logarithm: log 4 64
- Let's imagine the base and argument as a power of two: 4 = 2 2 ; 64 = 2 6 ;
- Let's create and solve the equation:
log 4 64 = b ⇒ (2 2) b = 2 6 ⇒ 2 2b = 2 6 ⇒ 2b = 6 ⇒ b = 3; - We received the answer: 3.
Task. Calculate the logarithm: log 16 1
- Let's imagine the base and argument as a power of two: 16 = 2 4 ; 1 = 2 0 ;
- Let's create and solve the equation:
log 16 1 = b ⇒ (2 4) b = 2 0 ⇒ 2 4b = 2 0 ⇒ 4b = 0 ⇒ b = 0; - We received the answer: 0.
Task. Calculate the logarithm: log 7 14
- Let's imagine the base and argument as a power of seven: 7 = 7 1 ; 14 cannot be represented as a power of seven, since 7 1< 14 < 7 2 ;
- From the previous paragraph it follows that the logarithm does not count;
- The answer is no change: log 7 14.
A small note to last example. How can you be sure that a number is not an exact power of another number? It’s very simple - just factor it into prime factors. And if such factors cannot be collected into powers with the same exponents, then the original number is not an exact power.
Task. Find out whether the numbers are exact powers: 8; 48; 81; 35; 14.
8 = 2 · 2 · 2 = 2 3 - exact degree, because there is only one multiplier;
48 = 6 · 8 = 3 · 2 · 2 · 2 · 2 = 3 · 2 4 - is not an exact power, since there are two factors: 3 and 2;
81 = 9 · 9 = 3 · 3 · 3 · 3 = 3 4 - exact degree;
35 = 7 · 5 - again not an exact power;
14 = 7 · 2 - again not an exact degree;
Let us also note that we ourselves prime numbers are always exact degrees of themselves.
Decimal logarithm
Some logarithms are so common that they have a special name and symbol.
The decimal logarithm of x is the base 10 logarithm, i.e. The power to which the number 10 must be raised to obtain the number x. Designation: lg x.
For example, log 10 = 1; lg 100 = 2; lg 1000 = 3 - etc.
From now on, when a phrase like “Find lg 0.01” appears in a textbook, know that this is not a typo. This is a decimal logarithm. However, if you are unfamiliar with this notation, you can always rewrite it:
log x = log 10 x
Everything that is true for ordinary logarithms is also true for decimal logarithms.
Natural logarithm
There is another logarithm that has its own designation. In some ways, it's even more important than decimal. We are talking about the natural logarithm.
The natural logarithm of x is the logarithm to base e, i.e. the power to which the number e must be raised to obtain the number x. Designation: ln x .
Many will ask: what is the number e? This is an irrational number; its exact value cannot be found and written down. I will give only the first figures:
e = 2.718281828459...
We will not go into detail about what this number is and why it is needed. Just remember that e is the base of the natural logarithm:
ln x = log e x
Thus ln e = 1; ln e 2 = 2; ln e 16 = 16 - etc. On the other hand, ln 2 is an irrational number. In general, the natural logarithm of any rational number irrational. Except, of course, for one: ln 1 = 0.
For natural logarithms all the rules that are true for ordinary logarithms are valid.
DEFINITION
Decimal logarithm called the base 10 logarithm:
Title="Rendered by QuickLaTeX.com">!}
This logarithm is the solution exponential equation. Sometimes (especially in foreign literature) the decimal logarithm is also denoted as , although the first two designations are also inherent in the natural logarithm.
The first tables of decimal logarithms were published by the English mathematician Henry Briggs (1561-1630) in 1617 (therefore, foreign scientists often call decimal logarithms still Briggs), but these tables contained errors. Based on the tables (1783) of the Slovenian and Austrian mathematician Georg Barthalomew Vega (Juri Veha or Vehovec, 1754-1802), in 1857 the German astronomer and surveyor Karl Bremiker (1804-1877) published the first error-free edition. With the participation of the Russian mathematician and teacher Leonty Filippovich Magnitsky (Telyatin or Telyashin, 1669-1739), the first tables of logarithms were published in Russia in 1703. Decimal logarithms were widely used for calculations.
Properties of Decimal Logarithms
This logarithm has all the properties inherent in a logarithm to an arbitrary base:
1. Basic logarithmic identity:
5. ![]() .
.
7. Transition to a new base:
![]()
The decimal logarithm function is a function. The graph of this curve is often called logarithmic.
Properties of the function y=lg x
1) Scope of definition: .
2) Multiple meanings: .
3) General function.
4) The function is non-periodic.
5) The graph of the function intersects the x-axis at point .
6) Intervals of constancy of sign: title="Rendered by QuickLaTeX.com" height="16" width="44" style="vertical-align: -4px;"> для !} ![]() that for .
that for .
They often take the number ten. Logarithms of numbers based on base ten are called decimal. When performing calculations with the decimal logarithm, it is common to operate with the sign lg, but not log; in this case, the number ten, which determines the base, is not indicated. Yes, let's replace log 10 105 to simplified lg105; A log 10 2 on lg2.
For decimal logarithms the same features that logarithms have with a base greater than one are typical. Namely, decimal logarithms are characterized exclusively for positive numbers. The decimal logarithms of numbers greater than one are positive, and those of numbers less than one are negative; of two non-negative numbers, the larger one is equivalent to the larger decimal logarithm, etc. Additionally, decimal logarithms have distinctive features and peculiar features that explain why it is comfortable to prefer the number ten as the base of logarithms.
Before examining these properties, let us familiarize ourselves with the following formulations.
Integer part of the decimal logarithm of a number A is called characteristic, and the fractional one is mantissa this logarithm.
Characteristics of the decimal logarithm of a number A is indicated as , and the mantissa as (lg A}.
Let's take, say, log 2 ≈ 0.3010. Accordingly = 0, (log 2) ≈ 0.3010.
Likewise for log 543.1 ≈2.7349. Accordingly, = 2, (log 543.1)≈ 0.7349.
The calculation of decimal logarithms of positive numbers from tables is widely used.
Characteristic features of decimal logarithms.
The first sign of the decimal logarithm. not a whole negative number, represented by a one followed by zeros, is a positive integer equal to the number of zeros in the entry of the selected number .
Let's take log 100 = 2, log 1 00000 = 5.
Generally speaking, if
That A= 10n , from which we get
lg a = lg 10 n = n lg 10 =P.
Second sign. The ten logarithm of a positive decimal, shown as a one with leading zeros, is - P, Where P- the number of zeros in the representation of this number, taking into account zero integers.
Let's consider , log 0.001 = - 3, log 0.000001 = -6.
Generally speaking, if
,
That a= 10-n and it turns out
lga= lg 10n =-n log 10 =-n
Third sign. The characteristic of the decimal logarithm of a non-negative number greater than one is equal to the number of digits in the integer part of this number excluding one.
Let's analyze this feature: 1) The characteristic of the logarithm lg 75.631 is equal to 1.
Indeed, 10< 75,631 < 100. Из этого можно сделать вывод
lg 10< lg 75,631 < lg 100,
1 < lg 75,631 < 2.
This implies,
log 75.631 = 1 +b,
Comma offset in decimal to the right or to the left is equivalent to the operation of multiplying this fraction by a power of ten with an integer exponent P(positive or negative). And therefore, when the decimal point in a positive decimal fraction is shifted to the left or right, the mantissa of the decimal logarithm of this fraction does not change.
So, (log 0.0053) = (log 0.53) = (log 0.0000053).