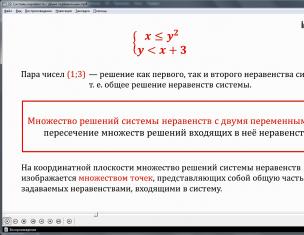
Consider the function given by the formula (equation)
This function, and therefore equation (11), corresponds to a well-defined line on the plane, which is the graph of this function (see Fig. 20). From the definition of the graph of a function it follows that this line consists of those and only those points of the plane whose coordinates satisfy equation (11).
Let it now
The line, which is the graph of this function, consists of those and only those points of the plane whose coordinates satisfy equation (12). This means that if a point lies on the specified line, then its coordinates satisfy equation (12). If the point does not lie on this line, then its coordinates do not satisfy equation (12).
Equation (12) is resolved with respect to y. Consider an equation containing x and y and not solved for y, such as the equation
Let us show that this equation in the plane also corresponds to a line, namely a circle with a center at the origin and a radius equal to 2. Let us rewrite the equation in the form
Its left side is the square of the distance of the point from the origin (see § 2, paragraph 2, formula 3). From equality (14) it follows that the square of this distance is equal to 4.
This means that any point whose coordinates satisfy equation (14), and therefore equation (13), is located at a distance of 2 from the origin.
The geometric location of such points is a circle with a center at the origin and radius 2. This circle will be the line corresponding to equation (13). The coordinates of any of its points obviously satisfy equation (13). If the point does not lie on the circle we found, then the square of its distance from the origin will be either greater or less than 4, which means that the coordinates of such a point do not satisfy equation (13).
Let now, in the general case, be given the equation
on the left side of which there is an expression containing x and y.
Definition. The line defined by equation (15) is the geometric locus of points in the plane whose coordinates satisfy this equation.
This means that if the line L is determined by an equation, then the coordinates of any point L satisfy this equation, but the coordinates of any point in the plane lying outside L do not satisfy equation (15).
Equation (15) is called the line equation
Comment. One should not think that any equation determines any line. For example, the equation does not define any line. In fact, for any real values of and y, the left side of this equation is positive and the right side is equal to zero, and therefore, this equation cannot be satisfied by the coordinates of any point in the plane
A line can be defined on a plane not only by an equation containing Cartesian coordinates, but also by an equation in polar coordinates. A line defined by an equation in polar coordinates is the geometric locus of points in the plane whose polar coordinates satisfy this equation.
Example 1. Construct an Archimedes spiral at .
Solution. Let's make a table for some values of the polar angle and the corresponding values of the polar radius.
We construct a point in the polar coordinate system, which obviously coincides with the pole; then, drawing the axis at an angle to the polar axis, we construct a point with a positive coordinate on this axis, after which we similarly construct points with positive values of the polar angle and polar radius (the axes for these points are not indicated in Fig. 30).
By connecting the points, we get one branch of the curve, indicated in Fig. 30 with a bold line. When changing from 0 to this branch of the curve consists of an infinite number of turns.
We will consider a line on a plane as the locus of points M(x, y) satisfying a certain condition.
If we write down in a Cartesian coordinate system a property that all points on a line have, connecting the coordinates and some constants, we can obtain an equation of the form: F(x, y) = 0 or .
Example. Write the equation of a circle with center at point C(x 0 , y 0) and radius R.
A circle is the geometric locus of points equidistant from point C. Let’s take point M with current coordinates. Then |CM| = R or 
or .
If the center of the circle is at the origin, then x 2 + y 2 = R 2 .
Not every equation of the form F(x, y) = 0 defines a line in the indicated sense: x 2 + y 2 = 0 is a point.
Straight on a plane.
Lines on a given plane are a special case of lines in space. Therefore, their equations can be obtained from the corresponding equations of lines in space.
General equation of a straight line on a plane. Equation of a straight line with an angular coefficient.
Any straight line in the XOY plane can be defined as the line of intersection of the Ax + By + Cz + D = 0 plane with the XOY plane: z = 0.
- straight line in the XOY plane: Ax + By + D = 0.
The resulting equation is called the general equation of the line. In the future we will write it in the form:
Ax + By + C = 0 (1)
1) Let , then or y = kx + b (2) – equation of a straight line with an angular coefficient. Let's find out the geometric meaning of k and b.
Let's put x = 0. Then y = b is the initial ordinate of the line.
Let's put y = 0. Then ; - slope coefficient of a straight line.
Special cases: a) b = 0, y=kx – the line passes through the origin; b) k = 0, y = b – straight line parallel to the OX axis; b) if B = 0, then Ax + C = 0, ,
This is the locus of points with constant abscissas equal to a, i.e. the straight line is perpendicular to the OX axis.
Equation of a straight line in segments.
Let the general equation of the line be given: Ax + By + C = 0, and . Let's divide both sides by –C:
or (3),
Where ; . This is the equation of a line in segments. Numbers a and b are the values of the segments cut off on the coordinate axes.
Equation of a line passing through a given point with a given slope.
Let a point M 0 (x 0 , y 0) lying on a straight line L and an angular coefficient k be given. Let's write the equation:
Here b is unknown. Let's find it, taking into account that M 0 L:
y 0 = kx 0 + b (**).
Subtract term by term from (1) (2):
y – y 0 = k(x – x 0) (4).
The equation of a line passing through a given point in a given direction.
Equation of a line passing through two given points.
Let two points M 1 (x 1 , y 1) and M 2 (x 2 , y 2) L be given. Let us write equation (4) in the form: y – y 1 = k(x – x 1). Because M 2 L, then y 2 – y 1 = k(x 2 – x 1). Let's divide it term by term:
(5),
This equation makes sense if , . If x 1 = x 2, then M 1 (x 1, y 1) and M 2 (x 1, y 2). If y 2 = y 1, then M 1 (x 1, y 1); M 2 (x 2, y 1).
Thus, if one of the denominators in (5) becomes zero, the corresponding numerator must be set equal to zero.
Example. M 1 (3, 1) and M 2 (-1, 4). Write the equation of the line passing through these points. Find k.
Let a Cartesian rectangular coordinate system Oxy and some line L be given on the plane.
Definition. Equation F(x;y)=0 (1) called equation of lineL(relative to a given coordinate system), if this equation is satisfied by the x and y coordinates of any point lying on the line L, and not by the x and y coordinates of any point not lying on the line L.
That. line on a plane is the locus of points (M(x;y)) whose coordinates satisfy equation (1).
Equation (1) defines the L line.
Example. Equation of a circle.
Circle– a set of points equidistant from a given point M 0 (x 0,y 0).
Point M 0 (x 0,y 0) – center of the circle.
For any point M(x;y) lying on the circle, the distance MM 0 =R (R=const)
MM 0 ==R
(x-x 0 ) 2 +(oooh 0 ) 2 =R 2 –(2) – equation of a circle of radius R with center at point M 0 (x 0,y 0).
Parametric equation of a line.
Let the x and y coordinates of points on line L be expressed using the parameter t:
(3) – parametric equation of the line in DSC
where the functions (t) and (t) are continuous with respect to the parameter t (in a certain range of variation of this parameter).
Excluding the parameter t from equation (3), we obtain equation (1).
Let us consider line L as the path traversed by a material point continuously moving according to a certain law. Let the variable t represent time counted from some initial moment. Then the specification of the law of motion represents the specification of the coordinates x and y of the moving point as some continuous functions x=(t) and y=(t) of time t.
Example. Let us derive a parametric equation for a circle of radius r>0 with center at the origin. Let M(x,y) be an arbitrary point of this circle, and t be the angle between the radius vector and the Ox axis, counted counterclockwise.
Then x=r cos x y=r sin t. (4)
Equations (4) are parametric equations of the circle under consideration. The parameter t can take any value, but in order for the point M(x,y) to go around the circle once, the range of the parameter change is limited to the half-segment 0t2.
By squaring and adding equations (4), we obtain the general equation of a circle (2).
2. Polar coordinate system (psc).
Let us choose the L axis ( polar axis) and determine the point of this axis O ( pole). Any point on the plane is uniquely defined by polar coordinates ρ and φ, where
ρ
– polar radius, equal to the distance from point M to pole O (ρ≥0);
φ – corner between vector direction OM and L axis ( polar angle). M(ρ ; φ )
Line equation in UCS can be written:
ρ=f(φ) (5) explicit equation of the line in the UCS
F=(ρ; φ) (6) implicit line equation in the UCS
Relationship between Cartesian and polar coordinates of a point.
(x;y)
(ρ ;
φ ) From triangle OMA:
tan φ=(restoration of the angleφ according to the knowntangent is producedtaking into account in which quadrant point M is located).(ρ ; φ )(x;y). x=ρcosφ,y=ρsinφ
Example . Find the polar coordinates of the points M(3;4) and P(1;-1).
For M:=5, φ=arctg (4/3). For P: ρ=; φ=Π+arctg(-1)=3Π/4.
Classification of flat lines.
Definition 1. The line is called algebraic, if in some Cartesian rectangular coordinate system, if it is defined by the equation F(x;y)=0 (1), in which the function F(x;y) is an algebraic polynomial.
Definition 2. Every non-algebraic line is called transcendental.
Definition 3. The algebraic line is called line of ordern, if in some Cartesian rectangular coordinate system this line is determined by equation (1), in which the function F(x;y) is an algebraic polynomial of the nth degree.
Thus, a line of nth order is a line defined in some Cartesian rectangular system by an algebraic equation of degree n with two unknowns.
The following theorem contributes to establishing the correctness of definitions 1,2,3.
Theorem(document on p. 107). If a line in some Cartesian rectangular coordinate system is determined by an algebraic equation of degree n, then this line in any other Cartesian rectangular coordinate system is determined by an algebraic equation of the same degree n.
1. Equation of a line on a plane
As you know, any point on the plane is determined by two coordinates in some coordinate system. Coordinate systems can be different depending on the choice of basis and origin.
Definition. The equation of a line is the relationship y = f (x) between the coordinates of the points that make up this line.
Note that the equation of a line can be expressed parametrically, that is, each coordinate of each point is expressed through some independent parameter t. A typical example is the trajectory of a moving point. In this case, the role of the parameter is played by time.
2. Equation of a straight line on a plane
Definition. Any straight line on the plane can be specified by a first-order equation Ax + By + C = 0, and the constants A, B are not equal to zero at the same time, i.e.
A 2 + B 2 ≠ 0. This first order equation is called the general equation of the line.
IN Depending on the values of constants A, B and C, the following special cases are possible:
– a straight line passes through the origin of coordinates
C = 0, A ≠ 0, B ≠ 0( By + C = 0) - straight line parallel to the Ox axis
B = 0, A ≠ 0, C ≠ 0( Ax + C = 0) – straight line parallel to the Oy axis
B = C = 0, A ≠ 0 – the straight line coincides with the Oy axis
A = C = 0, B ≠ 0 – the straight line coincides with the Ox axis
The equation of a straight line can be presented in different forms depending on any given initial conditions.
3. Equation of a straight line from a point and normal vector
Definition. In a Cartesian rectangular coordinate system, a vector with components (A, B) is perpendicular to the line given by the equation
Ax + By + C = 0.
Example. Find the equation of the line passing through the point A(1,2) perpendicular to the vector n (3, − 1).
With A=3 and B=-1, let’s compose the equation of the straight line: 3x − y + C = 0. To find the coefficient
Let us substitute the coordinates of the given point A into the resulting expression. We get: 3 − 2 + C = 0, therefore C = -1.
Total: the required equation: 3x − y − 1 = 0.
4. Equation of a line passing through two points
Let two points M1 (x1, y1, z1) and M2 (x2, y2, z2) be given in space, then the equation of the straight line is
passing through these points: |
x−x1 |
y−y1 |
z − z1 |
||||||||
− x |
− y |
− z |
|||||||||
If any of the denominators is zero, the corresponding numerator should be set equal to zero.
On the plane, the equation of the straight line written above is simplified: y − y 1 = y 2 − y 1 (x − x 1 ) if x 2 − x 1
x 1 ≠ x 2 and x = x 1 if x 1 = x 2 .
The fraction y 2 − y 1 = k is called the slope of the line. x 2 − x 1
5. Equation of a straight line using a point and slope
If the general equation of the straight line Ax + By + C = 0 is reduced to the form:
is called the equation of a straight line with slope k.
6. Equation of a straight line from a point and a direction vector
By analogy with the point considering the equation of a straight line through a normal vector, you can enter the definition of a straight line through a point and the directing vector of the straight line.
Definition. Each non-zero vector a (α 1 ,α 2 ) whose components satisfy the condition A α 1 + B α 2 = 0 is called a directing vector of the line
Ax + By + C = 0 .
Example. Find the equation of a straight line with a direction vector a (1,-1) and passing through the point A (1,2).
We will look for the equation of the desired line in the form: Ax + By + C = 0. In accordance with the definition, the coefficients must satisfy the conditions: 1A + (− 1) B = 0, i.e. A = B. Then the equation of the straight line has the form: Ax + Ay + C = 0, or x + y + C / A = 0. for x=1, y=2 we get C/A=-3, i.e. required equation: x + y − 3 = 0
7. Equation of a line in segments
If in the general equation of the straight line Ax + By + C = 0, C ≠ 0, then, dividing by –C,
we get: − |
x− |
y = 1 or |
1, where a = − |
b = − |
|||||||||
The geometric meaning of the coefficients is that coefficient a is the coordinate of the point of intersection of the line with the Ox axis, and b is the coordinate of the point of intersection of the line with the Oy axis.
8. Normal equation of a line
is called a normalizing factor, then we get x cosϕ + y sinϕ − p = 0 – the normal equation of the line.
The sign ± of the normalizing factor must be chosen so that μ C< 0 .
p is the length of the perpendicular lowered from the origin to the straight line, and ϕ is the angle formed by this perpendicular with the positive direction of the Ox axis
9. Angle between straight lines on a plane
Definition. If two lines are given y = k 1 x + b 1, y = k 2 x + b 2, then the acute angle between
Two lines are parallel if k 1 = k 2. Two lines are perpendicular if k 1 = − 1/ k 2 .
Equation of a line passing through a given point perpendicular to a given line
Definition. A straight line passing through point M1 (x1,y1) and perpendicular to the straight line y = kx + b is represented by the equation:
y − y = − |
(x − x) |
|||||||||||
10. Distance from a point to a line |
||||||||||||
If a point M(x0, y0) is given, then the distance to the straight line Ax + By + C = 0 |
||||||||||||
is defined as d = |
Ax0 + By0 + C |
|||||||||||
Example. Determine the angle between the lines: y = − 3x + 7, y = 2x + 1. |
||||||||||||
k = − 3, k |
2 tan ϕ = |
2 − (− 3) |
1;ϕ = π / 4. |
|||||||||
1− (− 3)2 |
||||||||||||
Example. Show, |
that the lines 3 x − 5 y + 7 = 0 and 10 x + 6 y − 3 = 0 |
|||||||||||
perpendicular. |
||||||||||||
We find: k 1 = 3/ 5, k 2 = − 5 / 3, k 1 k 2 = − 1, therefore, the lines are perpendicular.
Example. Given are the vertices of the triangle A(0; 1), B (6; 5), C (1 2; - 1).
Find the equation of the height drawn from vertex C. |
|||||||||||
Find the equation of side AB: |
x − 0 |
y − 1 |
y − 1 |
; 4x = 6 y − 6 |
|||||||
6 − 0 |
5 − 1 |
||||||||||
2 x − 3 y + 3 = 0; y = 2 3 x + 1.
The required height equation has the form: Ax + By + C = 0 or y = kx + bk = − 3 2 Then
y = − 3 2 x + b . Because height passes through point C, then its coordinates satisfy this equation: − 1 = − 3 2 12 + b, from where b=17. Total: y = − 3 2 x + 17.
Answer: 3x + 2 y − 34 = 0.
Equation of a line on a plane.
As is known, any point on the plane is determined by two coordinates in some coordinate system. Coordinate systems can be different depending on the choice of basis and origin.
Definition. Line equation called ratio y = f(x ) between the coordinates of the points that make up this line.
Note that the equation of a line can be expressed parametrically, that is, each coordinate of each point is expressed through some independent parametert.
A typical example is the trajectory of a moving point. In this case, the role of the parameter is played by time.
Equation of a straight line on a plane.
Definition. Any straight line on the plane can be specified by a first-order equation
Ax + Wu + C = 0,
Moreover, the constants A and B are not equal to zero at the same time, i.e. A 2 + B 2¹ 0. This first order equation is called general equation of a straight line.
Depending on the values of constants A, B and C, the following special cases are possible:
C = 0, A ¹ 0, B ¹ 0 – straight line passes through the origin
A = 0, B ¹ 0, C ¹ 0 ( By + C = 0) - straight line parallel to the Ox axis
B = 0, A ¹ 0, C ¹ 0 ( Ax + C = 0) – straight line parallel to the Oy axis
B = C = 0, A ¹ 0 – straight line coincides with the Oy axis
A = C = 0, B ¹ 0 – straight line coincides with the Ox axis
The equation of a straight line can be presented in different forms depending on any given initial conditions.
Distance from a point to a line.
Theorem. If a point M(x 0, y 0) is given, then the distance to the line Ax + Bу + C = 0 is determined as
.
Proof. Let point M 1 (x 1, y 1) be the base of a perpendicular dropped from point M to a given straight line. Then the distance between points M and M 1:
(1)
Coordinates x 1 and y 1 can be found as a solution to the system of equations:
The second equation of the system is the equation of a line passing through a given point M 0 perpendicular to a given line.
If we transform the first equation of the system to the form:
A(x – x 0) + B(y – y 0) + Ax 0 + By 0 + C = 0,
then, solving, we get:
Substituting these expressions into equation (1), we find:
.
The theorem has been proven.
Example. Determine the angle between straight lines: y = -3 x + 7; y = 2 x + 1.
K 1 = -3; k 2 = 2 tg j = ; j = p /4.
Example. Show that the lines 3x – 5y + 7 = 0 and 10x + 6y – 3 = 0 are perpendicular.
We find: k 1 = 3/5, k 2 = -5/3, k 1 k 2 = -1, therefore, the lines are perpendicular.
Example. Given the vertices of triangle A(0; 1), B (6; 5), C (12; -1). Find the equation of the height drawn from vertex C.
