VIII . Groups of construction tasks.
Solving groups of problems using an auxiliary triangle.
The essence of the method is the construction of auxiliary triangles and the use of their properties and newly obtained elements to finally solve the problem.
Construction analysis consists of the following steps:
Look for an auxiliary triangle in your analysis.
If new elements appear with the help of which it is possible to construct triangle ABC, then the goal has been achieved.
If this does not happen, then perhaps another auxiliary triangle can be constructed that will provide the missing elements.
Let's look at the essence of the method using examples.
Task 1. Construct an isosceles triangle ABC ( b= c) By a, h b .
We are looking for an auxiliary triangle. Obviously, it is convenient to consider triangle CDB as such a triangle.
This will give angle C, hence angle ABC. So, there is a, angle B, angle C, which means we can construct triangle ABC. We will write it schematically like this:
(a, h b) → Δ CDB →< C.
(a,< B, < C) → Δ ABC.
Tasks for independent decision:
Using reasoning similar to the above, we recommend constructing an isosceles triangle (b=c) using the following data:
A)< А, h b ;
b)< В, h с;
G)< В, h b ;
e)< С, h b .
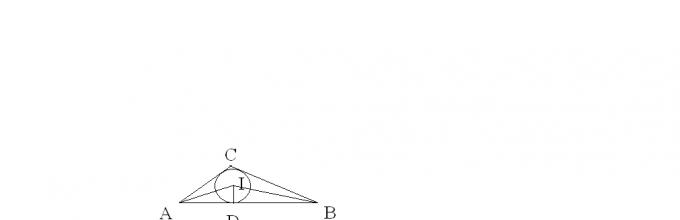
Task 2. Construct a triangle using the radius r of the inscribed circle, angle A and angle B.
Let I be the center of the circle inscribed in triangle ABC.
(r; ½< А) → Δ AID → |AD|;
(r; ½< В) → Δ ВID → |ВD|;
(|AD| + |ВD| = |AB|) → (c,< А, < В) → Δ ABC.
Tasks for independent solution:
Construct a triangle using the following elements:
a) a, h c, h b; b) a, h a, h b; c) a, m a, m b;
G)< A, l A , b; д) R, h а, m a ; е) a, R, h b ;
g) b, h b, m b (where m are medians, l are bisectors, h are heights).
On one's own:
Solving groups of problems based on the main one.
Main task:
construct a rhombus ABCD using diagonal BD and height BM. (ΔBHD →< BDH → равнобедренный Δ BDA → ABCD);
build a trapezoid on four sides.
Construct a triangle using two sides and the angle between them.
Main task:
Construct a right triangle along two sides.
Construct a rhombus along two diagonals.
Construct a rectangle with two unequal sides.
Construct a parallelogram using two diagonals and the angle between them.
Construct a rectangle using the diagonals and the angle between them.
Construct a triangle using a side and two adjacent angles.
Tasks for independent solution:
Main task:
Construct an isosceles triangle using its base and adjacent angle.
Construct a right triangle using a leg and an adjacent acute angle.
Construct a rhombus using an angle and a diagonal passing through the vertex of this angle.
Construct an isosceles triangle based on height and vertex angle.
Construct a square along the given diagonal.
Construct a right triangle using the hypotenuse and an acute angle.
Tasks for independent solution:
Main task:
Construct an isosceles triangle along the side and the corner at the base.
Construct an isosceles triangle using its side and vertex angle.
Construct a triangle using three sides.
Tasks for independent solution:
Main task:
Construct an isosceles triangle using its base and sides.
Construct a rhombus along the sides and diagonals.
Construct a parallelogram using two unequal sides and a diagonal.
Construct a parallelogram using a side and two diagonals.
Construct a right triangle using a leg and a hypotenuse.
Tasks for independent solution:
Construct an isosceles triangle along the height and side.
Construct an isosceles triangle using the base and a perpendicular from the end of the base to the side.
Construct a parallelogram using its base, height and diagonal.
Construct a rhombus along its height and diagonal.
Construct an isosceles triangle using the side and the height lowered from it.
Construct a triangle using its base, height and side.
Literature:
B. I. Argunov, M. B. Balk “Geometric constructions on the plane”, M, “Prosveshchenie” 1955.
Glazer G.I. “History of mathematics in school” IV – VI grades, M, “Enlightenment”, 1981
I. Goldenblant “Experience in solving geometric construction problems” “Mathematics at school” No. 3, 1946
I. A. Kushnir “On one way to solve construction problems” “Mathematics at school” No. 2, 1984
A. I. Mostovoy “Apply various methods of solving construction problems” “Mathematics at school” No. 5, 1983
A. A. Popova “Mathematics” Textbook. “Chelyabinsk State pedagogical university”, 2005
E. M. Selezneva, M. N. Serebryakova “ Geometric constructions in grades I – V high school“Methodological developments. Sverdlovsk, 1974
Isosceles is like this triangle, in which the lengths of its two sides are equal to each other.
When solving problems on the topic "Isosceles Triangle" it is necessary to use the following known properties:
1.
Angles opposite equal sides are equal to each other.
2.
Bisectors, medians and altitudes drawn from equal angles, are equal to each other.
3.
The bisector, median and altitude drawn to the base of an isosceles triangle coincide with each other.
4.
The center of the incircle and the center of the circumcircle lie at the height, and therefore at the median and bisector drawn to the base.
5.
Angles that are equal in an isosceles triangle are always acute.
A triangle is isosceles if it has the following signs:
1.
Two angles of a triangle are equal.
2.
The height coincides with the median.
3.
The bisector coincides with the median.
4.
The height coincides with the bisector.
5.
The two altitudes of a triangle are equal.
6.
The two bisectors of a triangle are equal.
7.
The two medians of a triangle are equal.
Let's consider several problems on the topic "Isosceles Triangle" and give their detailed solution.
Task 1.
In an isosceles triangle, the altitude to the base is 8, and the base to the side is 6:5. Find the distance from the vertex of the triangle to the point of intersection of its bisectors.
Solution.
Let an isosceles triangle ABC be given (Fig. 1).
1) Since AC: BC = 6: 5, then AC = 6x and BC = 5x. VN – height drawn to the base of the speaker triangle ABC.
Since point H is the middle of AC (according to the property of an isosceles triangle), then HC = 1/2 AC = 1/2 6x = 3x.
BC 2 = VN 2 + NS 2;
(5x) 2 = 8 2 + (3x) 2 ;
x = 2, then
AC = 6x = 6 2 = 12 and
BC = 5x = 5 2 = 10.
3) Since the point of intersection of the bisectors of a triangle is the center of the circle inscribed in it, then
OH = r. We find the radius of the circle inscribed in triangle ABC using the formula
4) S ABC = 1/2 · (AC · BH); S ABC = 1/2 · (12 · 8) = 48;
p = 1/2 (AB + BC + AC); p = 1/2 · (10 + 10 + 12) = 16, then OH = r = 48/16 = 3.
Hence VO = VN – OH; VO = 8 – 3 = 5.
Answer: 5.
Task 2.
In an isosceles triangle ABC, the bisector AD is drawn. The areas of triangles ABD and ADC are 10 and 12. Find the tripled area of a square constructed at the height of this triangle drawn to the base AC.
Solution.
Consider triangle ABC - isosceles, AD - bisector of angle A (Fig. 2).
1) Let us write down the areas of triangles BAD and DAC:
S BAD = 1/2 · AB · AD · sin α; S DAC = 1/2 · AC · AD · sin α.
2) Find the ratio of areas:
S BAD /S DAC = (1/2 · AB · AD · sin α) / (1/2 · AC · AD · sin α) = AB/AC.
Since S BAD = 10, S DAC = 12, then 10/12 = AB/AC;
AB/AC = 5/6, then let AB = 5x and AC = 6x.
AN = 1/2 AC = 1/2 · 6x = 3x.
3) From the triangle ABN - rectangular according to the Pythagorean theorem AB 2 = AN 2 + BH 2;
25x 2 = VN 2 + 9x 2;
4) S A ВС = 1/2 · АС · ВН; S A B C = 1/2 · 6x · 4x = 12x 2 .
Since S A BC = S BAD + S DAC = 10 + 12 = 22, then 22 = 12x 2 ;
x 2 = 11/6; VN 2 = 16x 2 = 16 11/6 = 1/3 8 11 = 88/3.
5) The area of the square is equal to VN 2 = 88/3; 3 88/3 = 88.
Answer: 88.
Task 3.
In an isosceles triangle, the base is 4 and the side is 8. Find the square of the height dropped to the side.
Solution.
In triangle ABC - isosceles BC = 8, AC = 4 (Fig. 3).
1) ВН – height drawn to the base AC of triangle ABC.
Since point H is the middle of AC (according to the property of an isosceles triangle), then HC = 1/2 AC = 1/2 4 = 2.
2) From the triangle VNS - rectangular according to the Pythagorean theorem BC 2 = VN 2 + NS 2;
64 = VN 2 + 4;
3) S ABC = 1/2 · (AC · BH), as well as S ABC = 1/2 · (AM · BC), then we equate the right-hand sides of the formulas, we get
1/2 · AC · BH = 1/2 · AM · BC;
AM = (AC BH)/BC;
AM = (√60 · 4)/8 = (2√15 · 4)/8 = √15.
Answer: 15.
Task 4.
In an isosceles triangle, the base and the height dropped onto it are equal to 16. Find the radius of the circle circumscribed about this triangle.
Solution.
In triangle ABC – isosceles base AC = 16, ВН = 16 – height drawn to the base AC (Fig. 4).
1) AN = NS = 8 (according to the property of an isosceles triangle).
2) From the VNS triangle - rectangular according to the Pythagorean theorem
BC 2 = VN 2 + NS 2;
BC 2 = 8 2 + 16 2 = (8 2) 2 + 8 2 = 8 2 4 + 8 2 = 8 2 5;
3) Consider triangle ABC: by the theorem of sines 2R = AB/sin C, where R is the radius of the circle circumscribed about triangle ABC.
sin C = BH/BC (from the triangle VNS by definition of sine).
sin C = 16/(8√5) = 2/√5, then 2R = 8√5/(2/√5);
2R = (8√5 · √5)/2; R = 10.
Answer: 10.
Task 5.
The length of the altitude drawn to the base of an isosceles triangle is 36, and the radius of the inscribed circle is 10. Find the area of the triangle.
Solution.
Let an isosceles triangle ABC be given.
1) Since the center of a circle inscribed in a triangle is the intersection point of its bisectors, then O ϵ VN and AO is the bisector of angle A, and also OH = r = 10 (Fig. 5).
2) VO = VN – OH; VO = 36 – 10 = 26.
3) Consider triangle ABN. By the theorem on the angle bisector of a triangle
AB/AN = VO/OH;
AB/AN = 26/10 = 13/5, then let AB = 13x and AN = 5x.
According to the Pythagorean theorem, AB 2 = AN 2 + VN 2;
(13x) 2 = 36 2 + (5x) 2 ;
169x 2 = 25x 2 + 36 2;
144x 2 = (12 · 3) 2 ;
144x2 = 144 9;
x = 3, then AC = 2 · AN = 10x = 10 · 3 = 30.
4) S ABC = 1/2 · (AC · BH); S ABC = 1/2 · (36 · 30) = 540;
Answer: 540.
Task 6.
In an isosceles triangle, two sides are equal to 5 and 20. Find the bisector of the angle at the base of the triangle.
Solution.
1) Suppose that the sides of the triangle are 5 and the base is 20.
Then 5 + 5< 20, т.е. такого треугольника не существует. Значит, АВ = ВС = 20, АС = 5 (Fig. 6).
2) Let LC = x, then BL = 20 – x. By the theorem on the angle bisector of a triangle
AB/AC = BL/LC;
20/5 = (20 – x)/x,
then 4x = 20 – x;
Thus LC = 4; BL = 20 – 4 = 16.
3) Let’s use the formula for the bisector of a triangle angle:
AL 2 = AB AC – BL LC,
then AL 2 = 20 5 – 4 16 = 36;
Answer: 6.
Still have questions? Don't know how to solve geometry problems?
To get help from a tutor, register.
The first lesson is free!
website, when copying material in full or in part, a link to the source is required.
How to construct an isosceles triangle? This is easy to do with a ruler, pencil and notebook cells.
We begin the construction of an isosceles triangle from the base. To make the pattern even, the number of cells at the base must be an even number.
Divide the segment - the base of the triangle - in half.
The vertex of the triangle can be chosen at any height from the base, but always exactly above the middle.
How to construct an acute isosceles triangle?
The angles at the base of an isosceles triangle can only be acute. In order for an isosceles triangle to be acute, the angle at the vertex must also be acute.
To do this, select the vertex of the triangle higher, away from the base.
The higher the apex, the smaller the apex angle. The angles at the base increase accordingly.
How to construct an obtuse isosceles triangle?
As the vertex of an isosceles triangle approaches the base degree measure the apex angle increases.
This means that in order to construct an isosceles obtuse triangle, choose a lower vertex.
How to construct an isosceles right triangle?
To construct an isosceles right triangle, you need to select a vertex at a distance equal to half the base (this is due to the properties of an isosceles right triangle).
For example, if the length of the base is 6 cells, then we place the vertex of the triangle at a height of 3 cells above the middle of the base. Please note: in this case, each cell at the corners at the base is divided diagonally.
The construction of an isosceles right triangle can be started from the vertex.
We select a vertex, and from it at right angles we lay equal segments up and to the right. These are the sides of the triangle.
Let's connect them and get an isosceles right triangle.
We will consider the construction of an isosceles triangle using a compass and a ruler without divisions in another topic.
